The ability of eucariotic cells to adopt a variety of shapes, organize the many components in their interior, interact mechanically with the environment, and carry out coordinated movements depends on the cytoskeleton – an intricate network of protein filaments that extends throughout the cytoplasm. This filamentous architecture helps to support the large volume of cytoplasm in a eukaryotic cell, a function that is particularly important in animal cells, which have no cell walls. Although some cytoskeletal components are present in bacteria, the cytoskeleton in the large and structurally complex eucariotic cell.
In our research we model the cells’ cytoskeleton with coarse-grained networks of polymers, i.e. long macromolecules connected together by crosslinking particle units. Here, atomistic simulations of protein fibers are not feasible when considering systems that have the size of a whole cell, i.e. which are of the size of several dozen micrometers. The cytoskeleton of real eucariotic cells is built on a framework of three types of protein filaments: intermediate filaments, microtubules and actin filamentswith all vastly different mechanical properties expressed in their persistence lengths Lp; each of the protein filaments is formed from a different protein subunit.
A family of fibrous proteins form the intermediate filaments; tubulin is the subunit in microtubules and actin is the subunit in actin filaments. In each case, thousands of subunits assemble into a fine thread of protein that sometimes extends across the entire cell. The differences in flexibility that exist among the three filament systems have been described qualitatively by comparing electron micrographs of negatively stained dehydrated filaments and by directly measuring the persistence length of intermediate filaments (100-200 nm), F-actin filaments (3–20 μm) and microtubules (1–8 mm) by various physical methods. For comparison, DNA, the largest naturally occuring polymer, exhibits a persistence length of Lp= 50 nm. In principle, in our simulations, we can take into account the different persistence lengths of these different types of meshed filaments by introducing semiflexibility into our polymer chains as is described further here.
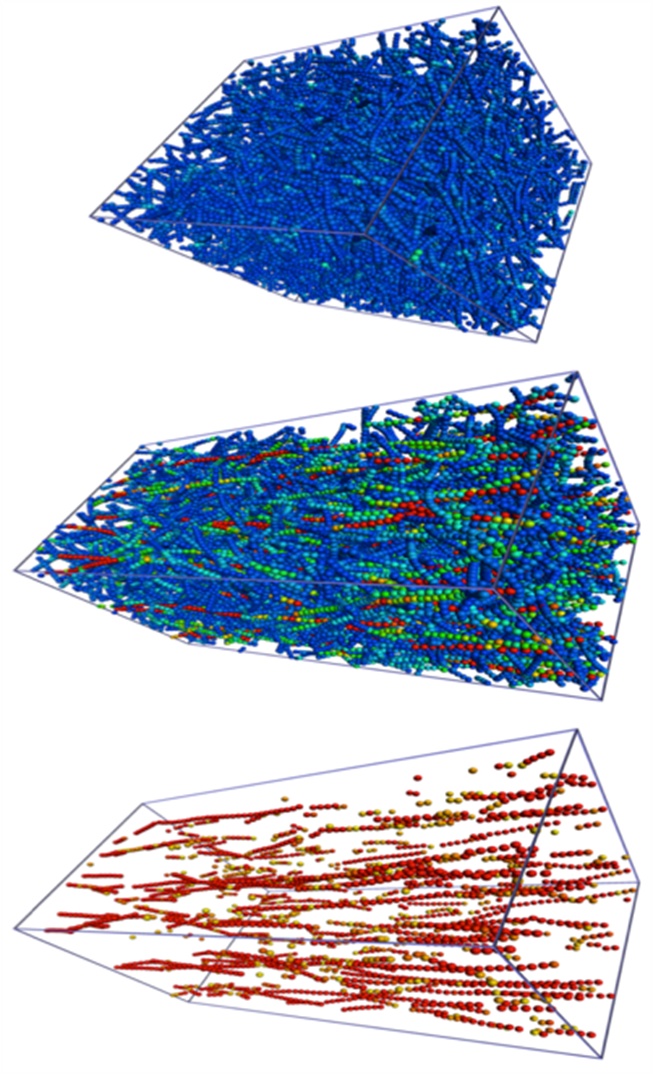
The simulation snapshots in Figure 1 show a simulation of a polymer network, where we apply tensile forces on both sides, thus stretching the network. From measurements of the resulting stress-strain curves we find a strongly non-linear, non-affine behavior of the polymer network upon stress loading which is also observed in measurements of in-vitro protein networks.